Reddening
Recent studies on high redshift galaxies and star formation obscured by
dust have shown the importance of the reddening in the high-z universe.
Therefore, another effect that must be taken into account is dust extinction,
produced inside the galaxies themselves.
The five reddening laws presently implemented in hyperz are:
-
Allen (1976) for the Milky Way (MW);
-
Seaton (1979) fit by Fitzpatrick (1986) for the MW;
-
Fitzpatrick (1986) for the Large Magellanic Cloud (LMC);
-
Prévot et al. (1984) and Bouchet et al. (1985) for the Small Magellanic
Cloud (SMC);
-
Calzetti et al. (2000) for starburst galaxies.
The different laws normalized to 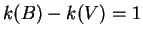 ,
are presented in Figure 4. The input value
is
,
are presented in Figure 4. The input value
is 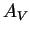 ,
corresponding to a dust-screen model, with
,
corresponding to a dust-screen model, with
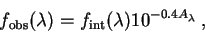
where 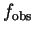 and
and 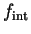 are the observed and the intrinsic fluxes, respectively. The extinction
at a wavelength
are the observed and the intrinsic fluxes, respectively. The extinction
at a wavelength 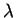 is related to the colour excess
is related to the colour excess 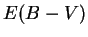 and to the reddening curve
and to the reddening curve 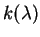 by
by
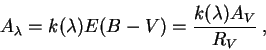
with 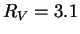 except for the Small Magellanic Cloud (
except for the Small Magellanic Cloud ( )
and Calzetti's law (
)
and Calzetti's law ( ).
).
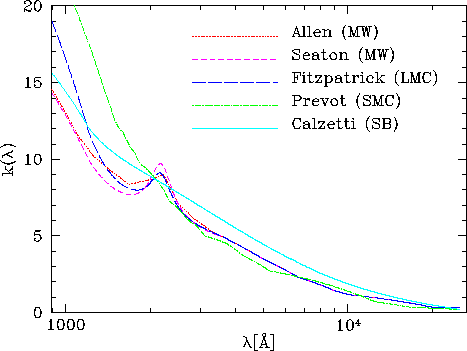 |
Figure:Extinction curves 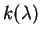 for the different reddening laws implemented in hyperz.
for the different reddening laws implemented in hyperz.
In detail, Allen's law for the MW is computed from the values of 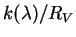 tabulated in Table 3. At shorter
and longer wavelengths we extrapolate the slope from the last available
points. For Seaton's law, we adopt the fit presented by Fitzpatrick (1986),
that follows the same relation found by the same author for the LMC:
tabulated in Table 3. At shorter
and longer wavelengths we extrapolate the slope from the last available
points. For Seaton's law, we adopt the fit presented by Fitzpatrick (1986),
that follows the same relation found by the same author for the LMC:
with  in
in 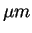 .
The two laws, for the MW and the LMC, are different in the coefficient
values:
.
The two laws, for the MW and the LMC, are different in the coefficient
values:
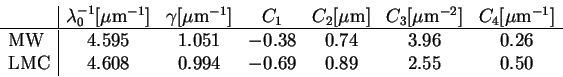
whereas 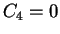 for
for 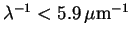 in any case. Because the validity of these laws is limited to
in any case. Because the validity of these laws is limited to 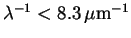 ,
i.e.
,
i.e. 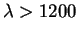 Å,
we extrapolated the slope at shorter wavelengths from the 1100 - 1200Å
range. At wavelengths longer than 3650 and 3330Å respectively for
the two quoted laws, we adopt the points from Allen, to avoid
Å,
we extrapolated the slope at shorter wavelengths from the 1100 - 1200Å
range. At wavelengths longer than 3650 and 3330Å respectively for
the two quoted laws, we adopt the points from Allen, to avoid 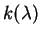 being too flat in the red and near-IR regions.
being too flat in the red and near-IR regions.
0.15cm
Table:Left: Values of 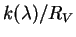 for the MW reddening law from Allen (1976). Right: Values of
for the MW reddening law from Allen (1976). Right: Values of 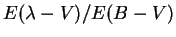 for the SMC reddening law from Prévot et al. (1984) and Bouchet
et al. (1985).
for the SMC reddening law from Prévot et al. (1984) and Bouchet
et al. (1985).
 [Å] [Å] |
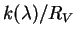 |
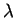 [Å] [Å] |
 |
| 1000. |
4.20 |
3650. |
1.58 |
| 1110. |
3.70 |
4000. |
1.45 |
| 1250. |
3.30 |
4400. |
1.32 |
| 1430. |
3.00 |
5000. |
1.13 |
| 1670. |
2.70 |
5530. |
1.00 |
| 2000. |
2.80 |
6700. |
0.74 |
| 2220. |
2.90 |
9000. |
0.46 |
| 2500. |
2.30 |
10000. |
0.38 |
| 2850. |
1.97 |
20000. |
0.11 |
| 3330. |
1.69 |
100000. |
0.00 |
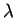 [Å] [Å] |
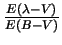 |
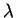 [Å] [Å] |
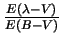 |
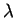 [Å] [Å] |
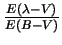 |
| 1275. |
13.54 |
1810. |
7.17 |
2778. |
3.15 |
| 1330. |
12.52 |
1860. |
6.90 |
2890. |
3.00 |
| 1385. |
11.51 |
1910. |
6.76 |
2995. |
2.65 |
| 1435. |
10.80 |
2000. |
6.38 |
3105. |
2.29 |
| 1490. |
9.84 |
2115. |
5.85 |
3704. |
1.81 |
| 1545. |
9.28 |
2220. |
5.30 |
4255. |
1.00 |
| 1595. |
9.06 |
2335. |
4.53 |
5291. |
0.00 |
| 1647. |
8.49 |
2445. |
4.24 |
12500. |
-2.02 |
| 1700. |
8.01 |
2550. |
3.91 |
16500. |
-2.36 |
| 1755. |
7.71 |
2665. |
3.49 |
22000. |
-2.47 |
|
The points used to compute the law for the SMC are taken from Prévot
et al. (1984) and Bouchet et al. (1985) and are listed in Table 3.
The peculiarity of this law is the lack of the graphite 2175Å bump.
The non detection of this bump is probably related to the large underabundance
of carbon in the SMC. As for Allen's law, we made an extrapolation from
the slope computed from the available points.
The most used attenuation curve in high-redshift studies is the law
derived by Calzetti et al. (2000). They derived a law as a purely empirical
result from a sample of near starburst (SB) galaxies. Again, the most prominent
feature of the MW law is absent: this characteristic suggests that starburst
galaxies contain SMC-like dust grains, being the 2175Å bump an excellent
probe of the type of dust in a galaxy. From Calzetti et al. (2000) we have:
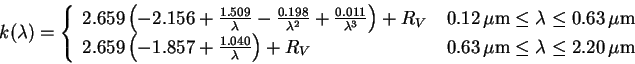
with 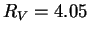 .
Below the validity wavelength range, we obtain the slope of the reddening
law by interpolating
.
Below the validity wavelength range, we obtain the slope of the reddening
law by interpolating 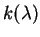 at 1100 and 1200Å. In a similar way, we compute the slope at
at 1100 and 1200Å. In a similar way, we compute the slope at 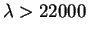 Å
from the values at 21900 and 22000Å.
Å
from the values at 21900 and 22000Å.
In particular, the application of this law is suggested for the central
star-forming regions of galaxies, and then for high-z galaxies. For this
reason, Calzetti's law is used to correct the value of the SFR at high-redshift,
as seen in the previous chapter. It is evident from Figure 4
that the UV region of the spectrum will be more affected by dust. As a
consequence, taking extinction into account is of paramount importance
for galaxies at 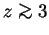 ,
when the rest frame UV emission is shifted to optical wavelengths.
,
when the rest frame UV emission is shifted to optical wavelengths.
Similarly, but in the opposite way, an extinction curve and a value
of the colour excess can be used to deredden the magnitudes of objects
seen through the dust of the Milky Way. see next section with parameters
description
micol bolzonella
2000-12-10
![]() and
and ![]() are the observed and the intrinsic fluxes, respectively. The extinction
at a wavelength
are the observed and the intrinsic fluxes, respectively. The extinction
at a wavelength ![]() is related to the colour excess
is related to the colour excess ![]() and to the reddening curve
and to the reddening curve ![]() by
by
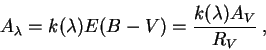
![]() except for the Small Magellanic Cloud (
except for the Small Magellanic Cloud (![]() )
and Calzetti's law (
)
and Calzetti's law (![]() ).
).

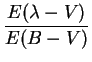
![$\displaystyle C_1 + C_2 \lambda^{-1} +\frac{C_3}{\left[\lambda^{-1}-\frac{(\lambda_0^{-1})^2}{\lambda^{-1}}\right] + \gamma^2}$](img93.gif)
![]() in
in ![]() .
The two laws, for the MW and the LMC, are different in the coefficient
values:
.
The two laws, for the MW and the LMC, are different in the coefficient
values:
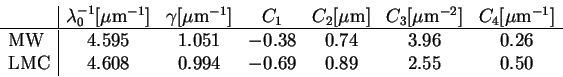
![]() for
for ![]() in any case. Because the validity of these laws is limited to
in any case. Because the validity of these laws is limited to ![]() ,
i.e.
,
i.e. ![]() Å,
we extrapolated the slope at shorter wavelengths from the 1100 - 1200Å
range. At wavelengths longer than 3650 and 3330Å respectively for
the two quoted laws, we adopt the points from Allen, to avoid
Å,
we extrapolated the slope at shorter wavelengths from the 1100 - 1200Å
range. At wavelengths longer than 3650 and 3330Å respectively for
the two quoted laws, we adopt the points from Allen, to avoid ![]() being too flat in the red and near-IR regions.
being too flat in the red and near-IR regions.
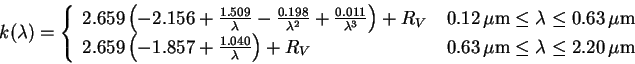
![]() .
Below the validity wavelength range, we obtain the slope of the reddening
law by interpolating
.
Below the validity wavelength range, we obtain the slope of the reddening
law by interpolating ![]() at 1100 and 1200Å. In a similar way, we compute the slope at
at 1100 and 1200Å. In a similar way, we compute the slope at ![]() Å
from the values at 21900 and 22000Å.
Å
from the values at 21900 and 22000Å.
![]() ,
when the rest frame UV emission is shifted to optical wavelengths.
,
when the rest frame UV emission is shifted to optical wavelengths.